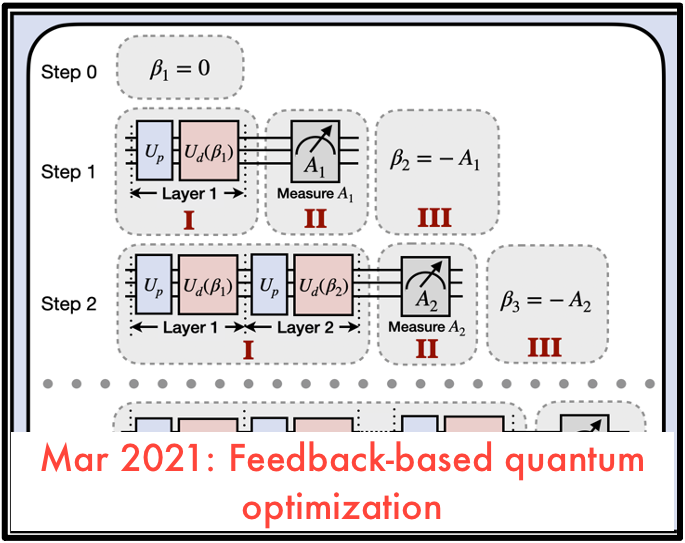
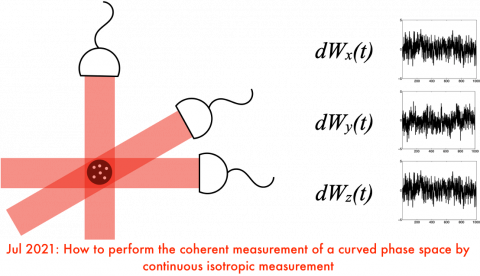
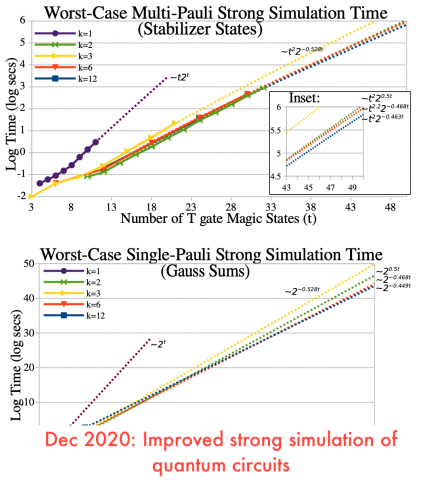
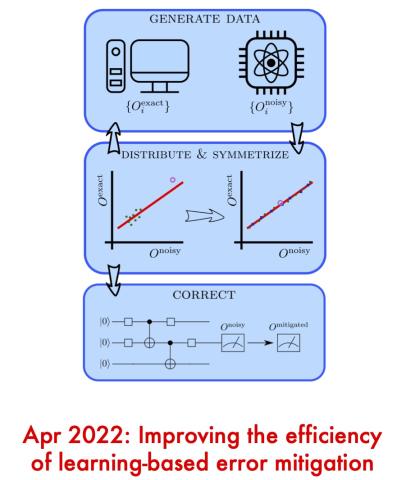
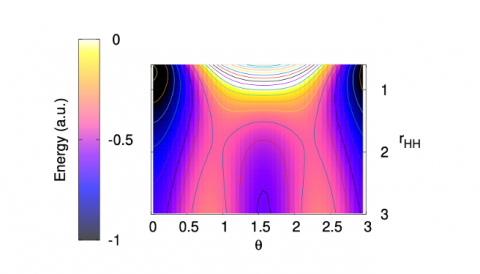
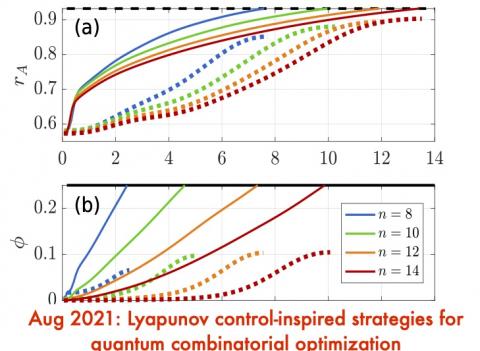
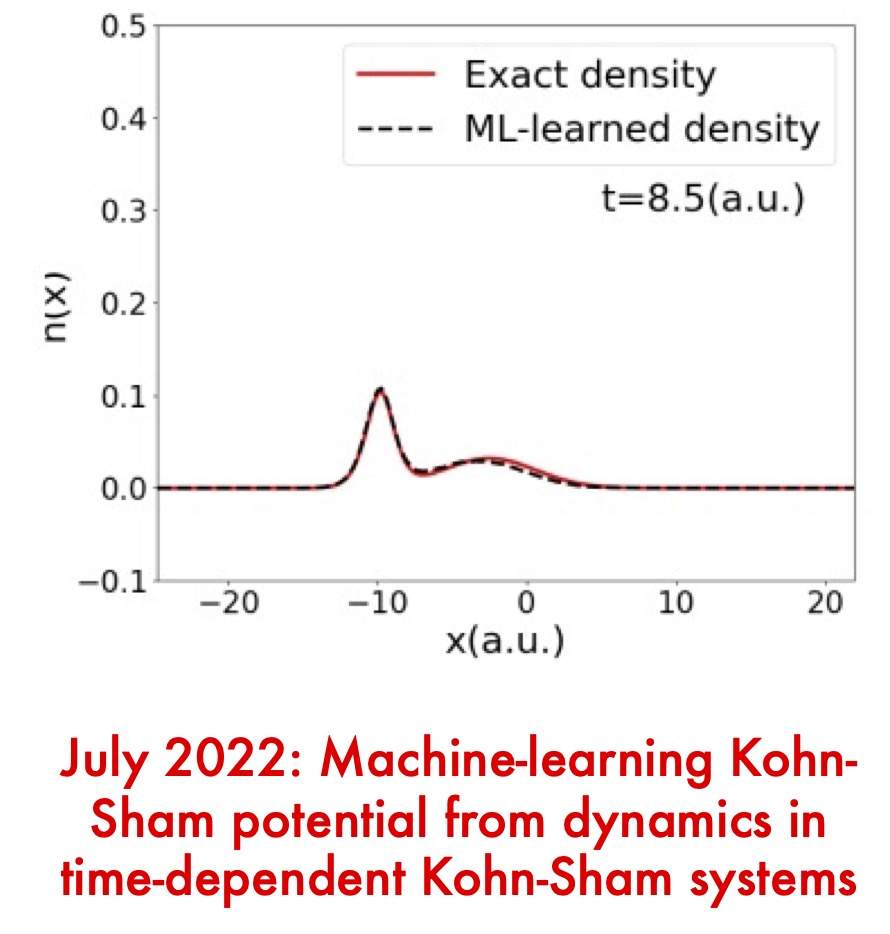
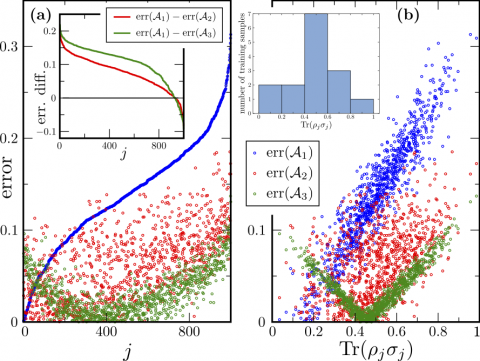
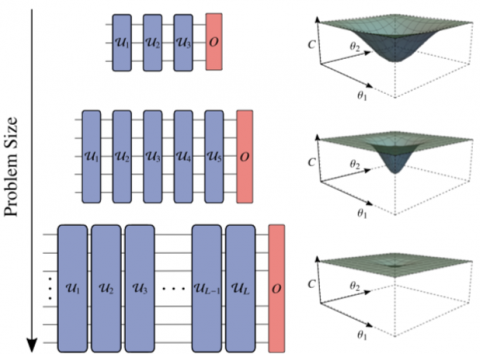
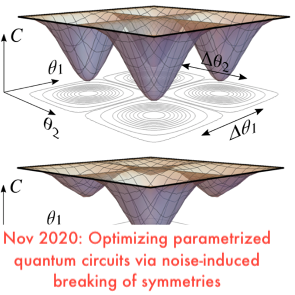
Post, September 19, 2020 • We provide an overview of variational quantum algorithms (VQAs) from the perspective of quantum optimal control (QOC). We integrate both fields in a common framework that enables identification of several QOC-inspired avenues for improving VQA performance on near-term quantum hardware. VQAs present some of the most promising applications of near-term...
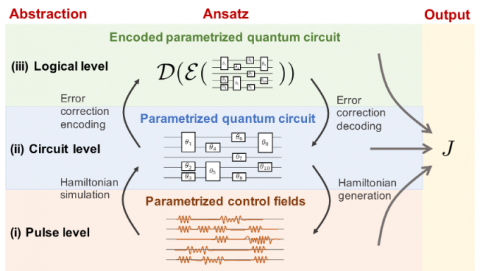
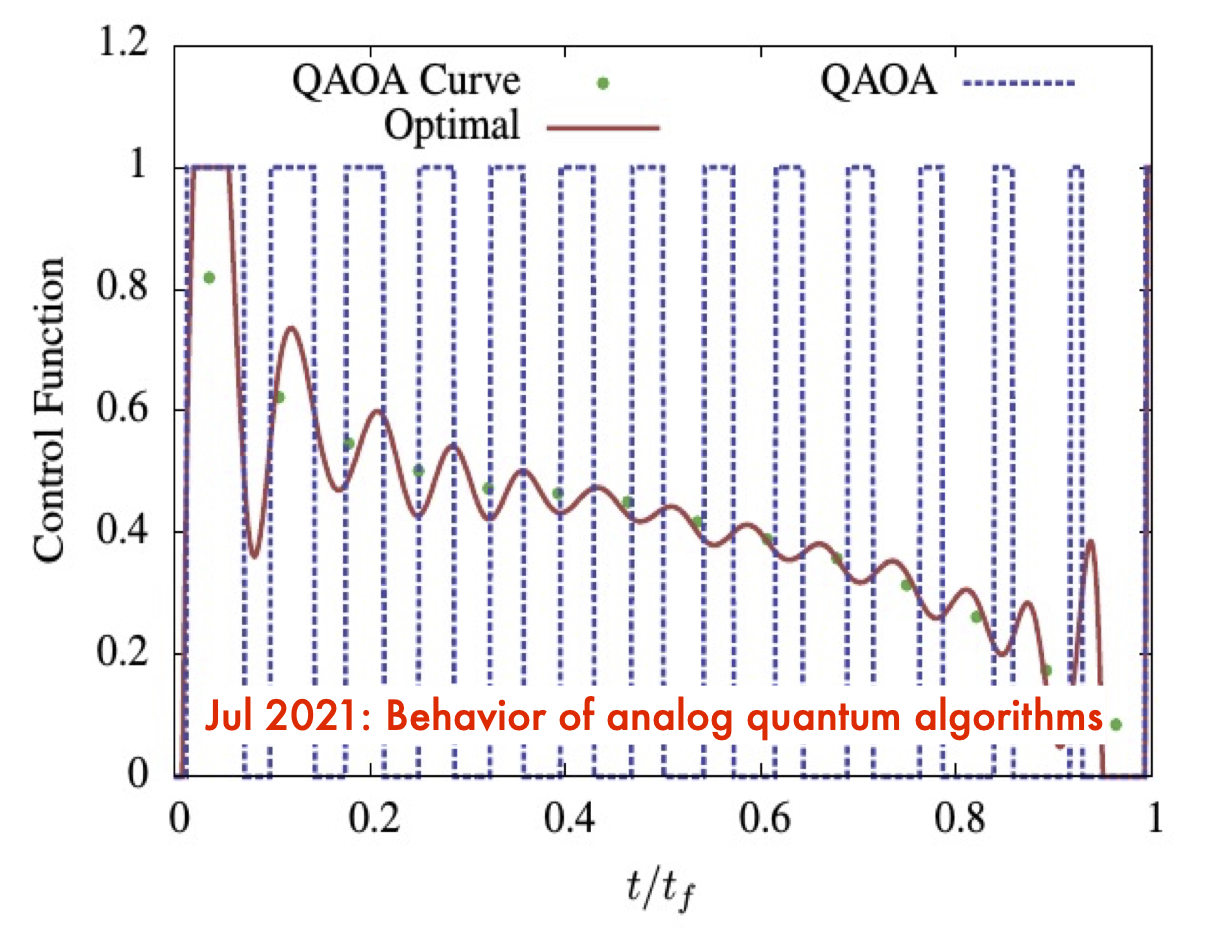
![The heavy-hexagon geometry as presented in [45]. 65 qubits are shown. (top right) We show a choice of grouping pairs of qubits together to encode the degree 3 vertices. (bottom) We show the system graph where each larger circle corresponds to one of the 49 encoded fermionic modes. Smaller circles represent the qubits associated to each mode.](https://www.sandia.gov/app/uploads/sites/216/2022/09/Picture1_5-1.png)